Home  -  Ueb Foundation  (Assessments)  -  Ueb Advanced  -  Nemeth  -  Use 6 Dot Entry

Set Notation

A Venn diagram is a visual representation of how sets of items (called members or elements) relate to each other.

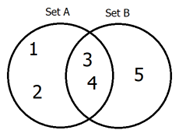
In set notation, each element of a set is listed inside brackets and special symbols are used to evaluate the sets.

Examples:

$Set\ A = \{ 1,\ 2,\ 3,\ 4\}$

⠠⠎⠑⠞⠀⠠⠁⠀⠐⠶⠀⠸⠣⠼⠁⠂⠀⠼⠃⠂⠀⠼⠉⠂⠀⠼⠙⠸⠜

$Set\ B = \{ 3,\ 4,\ 5\}$

⠠⠎⠑⠞⠀⠰⠠⠃⠀⠐⠶⠀⠸⠣⠼⠉⠂⠀⠼⠙⠂⠀⠼⠑⠸⠜

Signs of Operation for Set Notation

The intersection of two sets means the elements they have in common. The union of two sets means the elements of both sets combined. These symbols are signs of operation and do not require a space on either side of the symbol.

Intersection ∩	⠨⠦
Union ∪	⠨⠖

Examples:

$A\cap B=\{3,4\}$

⠠⠁⠨⠦⠠⠃⠀⠐⠶⠀⠸⠣⠼⠉⠂⠀⠼⠙⠸⠜

$A\cup B=\{1,2,3,4,5\}$

⠠⠁⠨⠖⠠⠃⠀⠐⠶⠀⠸⠣⠼⠁⠂⠀⠼⠃⠂⠀⠼⠉⠂⠀⠼⠙⠂⠀⠼⠑⠸⠜

Empty Set

When there are no elements in a set, it is called an empty set. This can be written as a set of opening and closing curly brackets with one visible empty space in between, or the symbol for null.

No elements - Empty Set { }	⠸⠣⠬⠸⠜
Null - Empty Set ∅	⠈⠚

Examples:

$B \cup D = \{\ \}$

⠠⠃⠨⠖⠠⠙⠀⠐⠶⠀⠸⠣⠬⠸⠜

$B \cup D = \ \varnothing\$

⠠⠃⠨⠖⠠⠙⠀⠐⠶⠀⠈⠚

Signs of Comparison for Set Notation

These symbols are signs of comparison and require a space on either side of the symbol.

Subset of/Includes ⊂	⠘⠣
Superset/Contains ⊃	⠘⠜
Element of, belongs to ∈	⠘⠑
Such That (vertical bar) |	⠳
Therefore ∴	⠠⠡
Since $\because$	⠈⠌
For Every ∀	⠘⠁
There exists ∃	⠘⠢
Drop (backslash) \	⠸⠡
Cancellation/not	⠈⠱

Examples:

$C\subset B$

⠰⠠⠉⠀⠘⠣⠀⠀⠰⠠⠃

$A\supset C$

⠠⠁⠀⠘⠜⠀⠰⠠⠉

$5\in B$

⠼⠑⠀⠘⠑⠀⠰⠠⠃

$4\in A^{\prime }\cap B^{\prime }$

⠼⠙⠀⠘⠑⠀⠰⠰⠠⠁⠶⠨⠦⠠⠃⠶

$\{x|x<5\}$

⠸⠣⠰⠭⠀⠸⠳⠀⠰⠭⠀⠈⠣⠀⠼⠑⠸⠜

$a=b\therefore b=a$

⠁⠀⠐⠶⠀⠰⠃⠀⠰⠠⠡⠀⠰⠃⠀⠐⠶⠀⠁

$\Rightarrow a=c(\because a=b)$

⠰⠳⠶⠕⠀⠁⠀⠐⠶⠀⠰⠉⠀⠐⠣⠈⠌⠀⠁⠀⠐⠶⠀⠰⠃⠐⠜

$\forall y\in Y$

⠘⠁⠀⠰⠽⠀⠘⠑⠀⠰⠠⠽

$\exists x:P\left(x\right)$

⠘⠢⠀⠭⠰⠒⠠⠏⠐⠣⠭⠐⠜

Negation

The symbol for negation can be used to express "not" with these signs of comparison. The line through previous item symbol can be used.

Examples:

$4\notin A$

⠼⠙⠀⠘⠑⠈⠱⠀⠠⠁

$X\not\subset Y$

⠰⠠⠭⠀⠘⠣⠈⠱⠀⠰⠠⠽

$C\ne 4$

⠰⠠⠉⠀⠐⠶⠈⠱⠀⠼⠙

Dropped Set or "Set Minus"

The backslash is used to show a dropped set, for example "belongs to C but not D."

Backslash

⠸⠡

Examples:

$A\backslash B=\{a\in A|a\notin B\}$

⠠⠁⠸⠡⠠⠃⠀⠐⠶⠀⠸⠣⠁⠀⠘⠑⠀⠠⠁⠀⠳⠸⠀⠁⠀⠘⠑⠈⠱⠀⠰⠠⠃⠸⠜

$\text{if}c=\{3,4\},\text{then}c\backslash d=3$

⠊⠋⠀⠰⠉⠀⠐⠶⠀⠸⠣⠼⠉⠂⠀⠼⠙⠸⠜⠀⠯⠀⠰⠙⠀⠐⠶⠀⠸⠣⠼⠙⠂⠀⠼⠑⠸⠜⠂⠀⠮⠝

⠉⠸⠡⠙⠀⠐⠶⠀⠼⠉

Embellished Letters

Various types of embellished letters are used in print to represent commons sets of numbers such as real numbers, integers, natural numbers, or universal set.

Examples: $\mathbb{R\ \ }\mathfrak{R\ \ }\mathcal{R}$

These embellishments are represented in braille by using the script typeform indicator.

Typeform indicator for script	⠈
Extent of indicator: next symbol	⠆

As you will remember, the typeform indicator is a two-cell indicator. The first cell indicates which type, the second cell tells what the typeform will apply to.

Examples:

$\mathbb{Q}=\text{rational numbers}$

⠈⠆⠰⠠⠟⠀⠐⠶⠀⠗⠁⠰⠝⠁⠇⠀⠝⠥⠍⠃⠻⠎

$\text{Set of integers}\mathbb{Z}$

⠠⠎⠑⠞⠀⠷⠀⠔⠞⠑⠛⠻⠎⠀⠈⠆⠰⠠⠵

$\mathbb{N}=\{1,2,3,\dots \}$

⠈⠆⠰⠠⠝⠀⠐⠶⠀⠸⠣⠼⠁⠂⠀⠼⠃⠂⠀⠼⠉⠂⠀⠲⠲⠲⠸⠜

More Examples:

All of the following examples are set notation that follow all previous learned braille rules.

$\frac{2}{5}\mathbb{\ \notin \ Z}$

⠼⠃⠌⠑⠀⠘⠑⠈⠱⠀⠈⠆⠰⠠⠵

Ordered pair

$(a,\ b)$

⠐⠣⠁⠂⠀⠰⠃⠐⠜

Interval notation

$\lbrack 5,\ \infty)$

⠨⠣⠼⠑⠂⠀⠼⠿⠐⠜

$( - \infty,\ a\rbrack\$

⠐⠣⠐⠤⠼⠿⠂⠀⠁⠨⠜

Set Builder notation

$F = \{ y^{2}:1\ \leq y\ \geq 10\}$

⠰⠠⠋⠀⠐⠶⠀⠸⠣⠽⠰⠔⠼⠃⠒⠼⠁⠀⠸⠈⠣⠀⠰⠽⠀⠸⠈⠜⠀⠼⠁⠚⠸⠜